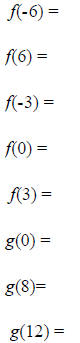Math 107 Exam 1 Practice
20. Use the graphs of f and g to evaluate the functions.
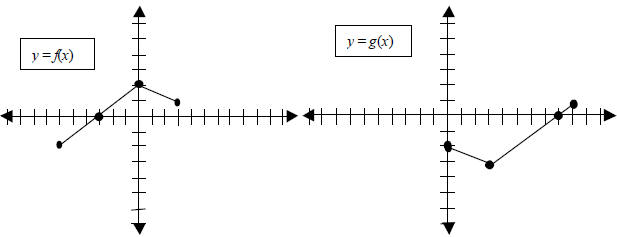
| Find:
|
21. Given the graph of f(x) on the axes below, find the
following.
(a) Find the domain of f(x): ___________________________
interval notation
(b) Find the range of f(x): _____________________________
interval notation
(c) Over which interval(s) is the function increasing?
___________________________
interval notation
(d) Over which interval(s) is the function decreasing?
___________________________
interval notation
(e) Over which interval(s) is the function constant?
_____________________________
interval notation
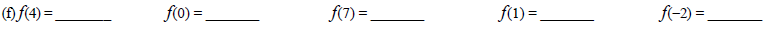
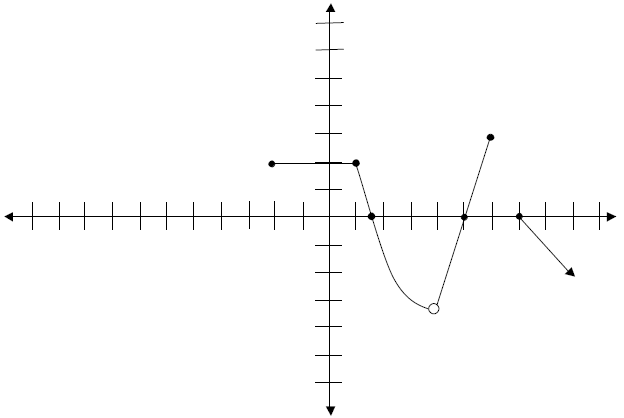
22. Using the function f(x) above (from #21), graph the
function y = -f(x + 6) + 3 on the same axes; label the points of the transformed
graph. Describe the transformation verbally.
23. Let f(x) = x2 - 25 and
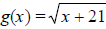. Find the following.
24. Find the mathematical model for the following: F
varies jointly as r and the cube of s. When F = 4158, r = 11 and s = 3.
Use the math model to find r, when F = 6 and s = 2.
25. C varies inversely as t and directly as z. If C = 10, when t = 2 and z = 12, then use a math model to find C, when t = 3 and z = 6.
26. Identify and graph the parent function for
.
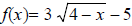Describe the transformation that occurs in the function, and then graph the
function.
Identify any intercepts.
Describe :
27. Identify and graph the parent function for
.
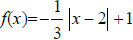Describe the transformation that occurs in the function, and then graph the
function.
Identify any intercepts.
Describe :
28. Given the quadratic function
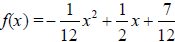, find the following. BOX YOUR ANSWERS.
(a) vertex
(b) y-intercept
(c) x-intercept(s)
(d) Standard Form
(d) Quadratic Form
29. Determine the Range of
in interval notation.
30. Find the difference quotient:
, where h ≠ 0, for each of the functions
31. A ball thrown into the air by a child follows a
parabolic path. The ball reaches a maximum height of 20 feet when it is 6 feet
away from
the child. If the ball is 5 feet off of the ground when the child releases the
ball, then how far from the child will the ball be when it hits the
ground? (Hint: Let y = f(x) be the vertical distance (height) of the ball and x
be the horizontal distance that the ball travels.)
Give an exact distance and an estimated distance (without using a calculator).
32. A machine producing toy trucks has a production time
of 10 hours before it conks out each day. Its production of toy trucks follows a
model of T(x) = -120x2 + 1200x, where x is the production time in hours and T(x)
is the number of toy trucks the machine produces.
At what time each day will the machine produce the maximum number of toy trucks
if the company starts the machine at 7:00 am daily?
33. Given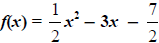 
, find the
following. Show your work when finding the vertex and the x-intercepts below.
Write NONE if that is the case. Sketch a graph of the function; label the vertex
and the x- and y-intercepts on the graph.
| (a) Equation in
Standard Form:
_______________________________ (b) vertex: _____________ (c) axis of symmetry: _____________ (d) opens: up or down (e) vertical: stretch or shrink or average (with respect to its parent graph) (f) Domain: _______________ (g) Range: ______________ (h) y-intercept: ____________ (i) x-intercept(s): _____________________ |
 |
34. Write the quadratic function
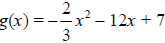in Standard Form.
35. Given the graph of h(x), graph the following
functions. Label the points that you graph clearly.
(8 points)
36. Identify and graph the parent function for
.
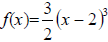Describe the transformation that occurs in the function, and then graph the
function.
Identify any intercepts.
Describe :
37. - 40. (Examples 1-4 in Section 2.7)