Multiplying Polynomials

I. Recall - 5⁴
A. The “5” is the base.
B. The “4” is the exponent.
C. The whole thing is called an exponential.
D. And 5⁴ = (5)(5)(5)(5) = 625.

II. Properties of Exponents
A. Adding
1. The exponent remains the same.
2. Examples
a. 2x + 3x = 5x
b. 10y³ – 15y³ = −5y³

B. Multiplying
1. Add the exponents of the things with the same bases.
2. Examples
a.
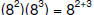
Answer: 8⁵

b.
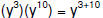
Answer: y¹³

c. Now you try one: (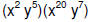
Answer:

C. Raising a power to a power
1. Multiply the exponents.
2. Examples
a.
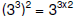
Answer: 3⁶

b.
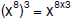
Answer: x²⁴

c. Now you try one:
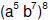
Answer:

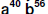
D. Power of a Product
1. “Distribute “ the exponent to all the factors.
a. (ab)⁵ = a⁵b⁵

2. Examples - Simplify each of the following.
a. (−6y)⁵
We need to raise both bases in the parentheses (-6 and y) to the fifth
power:
(−6)⁵ (y)⁵
OR
Answer: −7,776y⁵

b.
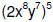
Again, we raise all factors to the fifth power:
OR
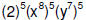Raise 2 to the 5^th; multiply the exponents on the variables.
Answer:

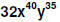
c. Now you try one: (2w²x³y)²
Answer:

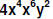
III. Multiplying Polynomials
A. When multiplying polynomials, use the distributive property. In other words, multiply
every term of one polynomial times every term of the other polynomial.

B. Remember that when you multiply like bases, you must multiply the coefficients and
add the exponents.

C. When possible, combine the like terms.

D. Examples – Multiply each of the following.

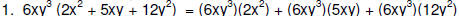
Answer:

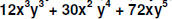
2. (x + 2)(x + 5) = (x)(x) + (x)(5) + (2)(x) + (2)(5) = x² + 5x + 2x + 10
Answer: x² + 7x + 10

3. Now you try one: (5x + 4)(3x + 2)
Answer: 15x² + 22x + 8

4. (1 – 3a)(3 + 2a) = (1)(3) + (1)(2a) + (-3a)(3) + (-3a)(2a) = 3 + 2a – 9a – 6a²
Answer: 3 – 7a – 6a²

5. Now you try one: (7 – 4x)(8 – 3x)
Answer: 56 – 53x + 12x²

6. (x – 2)(x² + 3x – 4) = (x)(x²) + (x)(3x) + (x)( −4) + (−2)(x²) + (−2)(3x) + (−2)( −4)
= x³ + 3x² – 4x – 2x² – 6x + 8
Answer: x³ + x² – 10x + 8

6a. You can also do this one vertically (as well as all the others):

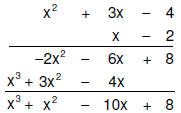
7. Now you try one: (a + 5)(a² + 2a + 3)
Answer: a³ + 7a² + 13a + 15

8. Find the area of the rectangle with dimensions 3x + 2 meters and x + 4
meters.
Let’s begin by drawing the figure:

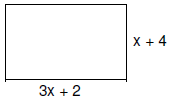
Remember that the formula for the area of a rectangle is A = LW. So we
have:
A = (3x + 2)(x + 4) = (3x)(x) + (3x)(4) + (2)(x)+ (2)(4)
OR
A = 3x² + 12x + 2x + 8

Answer: The area is 3x² + 14x + 8 square meters.

9. Now you try one: Find a trinomial for the area of the rectangular rug shown
in #101, page 340, whose sides are x + 5 feet and 2x – 3 feet.

Answer: 2x² + 7x – 15 square feet

10. Page 341, #104
a. Express the area of the large rectangle as the product of two binomials.

We notice that the length is in two parts; one part labeled 2x and one part
labeled 3. So the length will be what we get when we add the two parts.

Length = 2x + 3
The width is also in two parts. The width will be what we get when we add
those two parts.

Width = x + 2
The formula for the area of a rectangle is A = LW.

Answer: A = (2x + 3)(x + 2)

b. Find the sum of the areas of the four smaller rectangles.

The blue rectangle has a length of 2x and a width of x. When we multiply LW,
we get (2x)(x) = 2x².

The green rectangle has a length of 2x and a width of 2. When we multiply
LW, we get (2x)(2) = 4x.

The pink rectangle has a length of 3 and a width of x. When we multiply LW,
we get (3)(x) = 3x.

Finally, the tan rectangle has a length of 3 and a width of 2. When we multiply
LW, we get (3)(2) = 6.

Now adding up all of these pieces, we get:
A = 2x² + 4x + 3x + 6

Answer: A = 2x² + 7x + 6

c. Use polynomial multiplication to show that your expressions for area in parts
(a) and (b) are equal.

From part (a), we have:

A = (2x + 3)(x + 2) = 2x² + 4x + 3x + 6 = 2x² + 7x + 6

Which is the same as our answer from part (b).